|
Title:
The tesseract: visualising a whole new
world!
Author: Martin Drinkwater
Category: Cool Science
Many people consider 'dimensions' as a physical thing that
they can observe: a line, an irregular shape or a cube, for
instance. Having two eyes allows us to view an object from
two different angles, known as stereovision. This means that
we can learn to perceive depth from visual cues in three dimensions.
If you cover one eye and look at the world through only one
eye, you will only perceive a flat, two-dimensional world.
Most of
us are hard-pressed to imagine something that is 4 or even
5 dimensions because this is outside the area of our experience.
Mathematicians, however, think of dimensions as something
entirely different – an infinite number of dimensions can
exist. These dimensions are quite rigorously defined, which
has resulted in the assignment of fractal (fractional) dimensions
as well as whole number ones. All this 'dimensionalising'
can lead to theoretical structures with amazing and unusual
properties.
For instance,
think of a point. Project a point in one direction and you
have a line. Project a segment of this line into another dimension
perpendicular to the line – giving a total of 2 dimensions
– and you create a square or rectangle. Projecting a square
into another dimension results in a cube. Using this analogy,
we can construct figures with any number of dimensions, although
visualising them can lead to severe headaches!
The most
commonly studied higher-dimensional structure is the extension
of cube into another dimension, called a tesseract. There
is no mystery as to why the extension of the cube into further
dimensions is studied so much - this '4D' entity is the simplest
to visualise. Visualising is by no means an easy task: the
tesseract consists of 8 cubes, each restricted to the same
line segment on a single plane and 3 squares meeting at each
edge. The structure has a total of 24 faces, 16 vertices and
32 edges. You can create a shadowing effect by visualising
each cube in 3D space, and use your perceptual vision to see
the position of the others relative to it.
Confused?
To aid in the process, go to Alexander
Bogomolny's website and view the Java applet-based graphical
explanation. These images highlighting one of the cubes (in
three different orientations) come from that website.
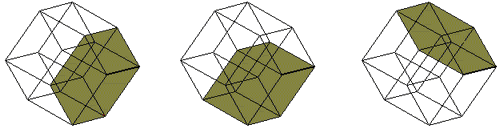
Potentially,
this process can be extended to an infinite number of dimensions.
The limiting factor is our ability to perceive these figures
or shapes and what we consider to be a ‘dimension’. For instance,
in physics, there are quantitative variables to which we have
assigned 'dimensions': metres, seconds, coulombs just to name
a few. Problems arise in that many of these are defined in
terms of other quantities - it can be argued that the Earth's
gravitational constant for example (6.67x10-11
m3/s2.kg) has 6 dimensions. These
quantities are dimensional in a different sense - they give
us different ways of looking at the geometric properties of
free space.
To complicate
things further, there are also 'dimensionless' quantities
related to angles such as the radian (the angle that one plane
makes with another plane) and steradian (the angle that one
solid makes against another). Scientists view these angles
as being dimensionless because they do not have an effect
on the construction of the object.
Our problem
lies with what the term ‘dimension’ is supposed to represent.
There is the use of dimensions to define variables and reduce
uncertainty, which is in contrast to our geometric interpretation
of space. They are mutually exclusive – one cannot be applied
to the other. So we can have infinitely many dimensions in
a theoretical context (when assigning variables), but we can
only perceive figures in these dimensions within
certain geometrical limits... Give me the good old squares
and cubes anyday!
For more
information see Alexander Bogomolny's "Generalizations
in Mathematics" at cut-the-knot.org
See OnSET's The
Magic Eye
|